Kohn anomalies in the phonon dispersion of single-walled carbon nanotubes (SWNTs)
|
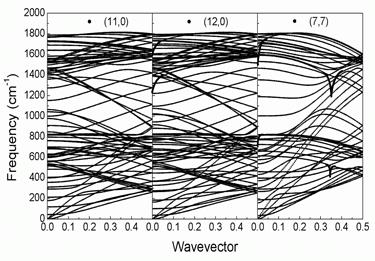
Fig. 1. Phonon
dispersion of three SWNTs calculated within a non-orthogonal tight-binding
model [1]. The phonon dispersion of the metallic SWNTs (12,0) and (7,7) shows
Kohn anomalies at the Brillouin zone center and inside the Brillouin zone due
to strong electron-phonon interactions [2], while that of the semiconducting
one (11,0) does not show such anomalies. |
The phonon dispersion of metallic SWNTs, calculated within the non-orthogonal tight-binding model and adopting the adiabatic approximation, shows Kohn anomalies for certain phonon branches at the zone center and inside the Brillouin zone, arising from strong scattering of electrons by phonons. Specifically, the Kohn anomalies are manifested by logarithmic singularities at electron wavevectors ±k* of electronic states, between which electrons are scattered by phonons with wavevectors ±q* with length q* = 2k* [2,3,4,5]. In particular, k* = 0 for zigzag SWNTs [3], k* = 1/3 for armchair SWNTs [4], and 0 < k* < 1/3 for chiral SWNTs [5] (Figs. 1 and 2). Additionally, the phonon dispersion of any metallic SWNT has anomalies at k* = 0. It is customary to derive the character of the phonon branches from those of graphene. Indeed, the phonon dispersion of any SWNT can be related to that of graphene by the zone-folding method. In this method, the unit cell of a SWNT is obtained by cutting out a rectangle from graphene and rolling it up into a seamless cylinder. The unit cell is repeated along the axis of the cylinder to form a one-dimensional periodic structure. The Brillouin zone of the SWNT is a rectangle as well. However, the periodic condition along the circumference of the SWNT restricts the allowed states to those on straight lines along the SWNT axis. These, so-called cutting lines, are labeled by the linear azimuthal quantum number, while the states along the cutting lines are labeled by the one-dimensional wavevector. The Kohn anomalies are enhanced for scattering of electrons by phonons in the case of strong electron-phonon coupling. For SWNTs, electrons are strongly coupled to optical phonons with in-plane longitudinal optical (LO) and in-plane transverse optical (TO) character. The electron-phonon coupling is especially strong for LO and TO phonons close to the Γ point, denoted here as LO(Γ) and TO(Γ), resp., as well as for TO phonons close to the K point, denoted as TO(K). The LO(Γ) and TO(Γ) branches have anomalies at the Γ point, and the TO(K) branch has an anomaly along the ΓK direction in the Brillouin zone (Figs. 2, 3, 4). Due to the strong electron-phonon coupling, corrections to the adiabatic LO(Γ), TO(Γ), and TO(K) branches, have to be evaluated (Piscanec). These, so-called non-adiabatic (or dynamic) corrections, are derived in first-order quantum-mechanical perturbation theory [5]. The resulting Kohn anomalies of the phonon branches are significantly modified and the phonon states get extra broadening ΔΓ (Figs. 2, 3, 4). In particular, the TO phonon at the Γ point does not change its frequency, while the frequency of the LO phonon is significantly upshifted (Fig. 4, left). The modification of the LO phonon frequency is most important for the assignment of the first-order Raman bands of SWNTs to particular nanotube types. The corrected LO(Γ) frequencies together with the previously derived TO(Γ) ones for metallic SWNTs, as well as the LO(Γ) and TO(Γ) frequencies for semiconducting nanotubes [6], are displayed in Fig. 6. (Note that, following the tradition, the LO(Γ) and TO(Γ) modes of metallic SWNTs are denoted as G−(M) and G+(M), while the LO(Γ) and TO(Γ) modes of semiconducting SWNTs denoted as G+(S) and G−(S)). The calculated LO(Γ) and TO(Γ) frequencies compare well to the available experimental data (open symbols: Paillet, Michel, Fouquet). The pair of lower frequency phonons agree with the ab-initio theoretical predictions (solid curves: Piscanec), while both theoretical sets are not in good agreement with the fits to the experimental data (dashed curves: Jorio). Our predictions for the LO(Γ) and TO(Γ) phonon frequencies were used for the assignment of Raman spectra of SWNTs [7,8,9]. References: 1. V. N. Popov and Ph. Lambin, Phys. Rev. B 73 (2006) 085407. 2. V. N. Popov and Ph. Lambin, Phys. Rev. B 74 (2006) 075415. 3. V. N. Popov, Physica E 44 (2010) 1032-1035. 4. V. N. Popov and Ph. Lambin, phys. stat. sol. (b) 247 (2010), 2784–2788. 5. V. N. Popov and Ph. Lambin, Nano Res. 3 (2010) 822–829. 6. V. N. Popov and Ph. Lambin, Phys. Rev. B 73 (2006) 085407. 7. D. I. Levshov et al., NanoLett. 11 (2011) 4800-4804. 8. H. N. Tran et al., Phys. Rev. B 94 (2016) 075430. 9. D. I. Levshov et al., Phys. Rev. B 96 (2017) 195410. |
|
Fig. 2. Phonon
dispersion of nanotube (8,5). Only phonon branches for cutting lines through
points Γ and K of the Brillouin zone of graphene are shown, while all
other branches are omitted. The branches for cutting lines through the Γ
point have anomalies at q* = 0 and for those through
the K point have anomalies at q* ≠ 0 [5]. |
|
|
Fig. 4. LO(Γ) and TO(Γ) branches (left) and
linewidth ΔΓ (right) for nanotube (8, 5) in the vicinity of the
Γ point. |
|
|
Fig. 5. TO(K) branch (left) and linewidth ΔΓ (right) of
nanotube (8,5) in the vicinity of the K point. |
|
|
Fig. 6. LO(Γ) and TO(Γ) phonon frequencies for all metallic
(M) and semiconducting (S) nanotubes with diameters d from 0.8 nm to 3.0 nm. The solid lines are DFT predictions (Piscanec). The dashed lines are fits to Raman data (Jorio). The open symbols are Raman data for individual nanotubes. Inset non-adiabatic broadening ΔΓ of the
LO(Γ) and TO(Γ) phonons for M nanotubes. |
Valentin Popov
November 21, 2010, May 11, 2018